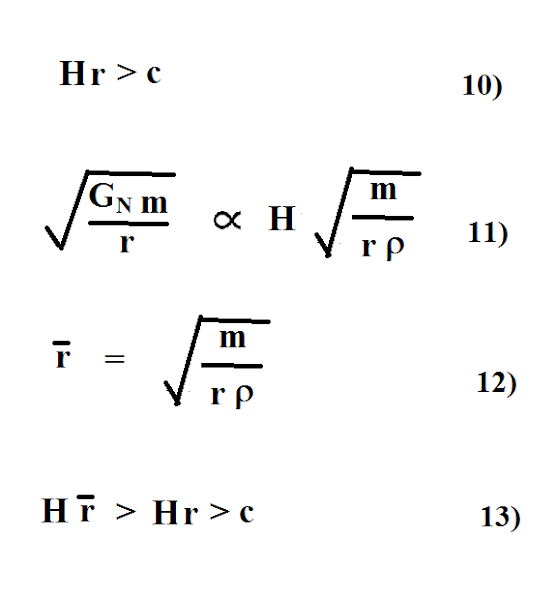Abstract:
This paper shows the connection between "dark energy" and gravity, the equivalence between matter and space, and how gravity works without the graviton or gravitational waves. It also suggests an alternate way to quantize gravity using smaller units--of mass, space and time--than the Planck units.
The same force acting on different masses will cause each mass to move at a different rate: F = ma, where m is mass and a is acceleration. However, the same "gravitational force" causes different masses to fall at the same rate. How can this be? Einstein proposed that when a body appears to be falling to earth, it is really at rest, and the earth is accelerating towards the body at a given rate. Thus the body's mass is irrelevant.
Below is a diagram of Alice who is surrounded by four bodies, including Bob. Bob and the others appear to be either moving away from Alice or towards her, depending on how you follow the arrows. But if we go from left to right, we can think of Alice as the one who is moving away from the surrounding bodies. If we go from right to left, we can think of Alice as the one who is moving towards the surrounding bodies. As a consequence, the surrounding bodies can have any mass and the rate at which Alice and the surrounding bodies diverge or converge will be the same.
Equations 1 and 2 below were derived from Einstein's field equations. Equation 3 was derived from a Friedmann equation where k is set to zero due to spacetime being flat at a large scale where the mass density (rho) is a small number and so is the curvature which is represented by the cosmological constant.
At 4 and 5 we set up a couple of substitutions to be made at 7 and 8 below. Equation 6 shows how mass and space are equivalent. Divide any mass by the vacuum-mass density to get the equivalent volume. Equation 7 shows the universe must expand faster than light as the radius r tends to infinity; otherwise, light speed in a vacuum is not conserved! If both sides of the equation are multiplied by the universe's mass, then the universe's energy is conserved no matter how big or small the universe becomes.
Also, the vacuum-mass density rho correlates with outward pressure, and that pressure is not diminished by an increase in distance r, so the outward pressure continues and so does the universe's expansion. At equation 8 we see that gravity looks similar to equation 7. As the speed of gravity increases, it is offset by the increase in spacetime curvature. As a result, the constant c and energy are conserved.
Equation 9 below shows that gravitational waves are caused by gravity and angular frequency, so they cannot be the cause of gravity. In fact, if angular frequency is zero, there is still gravity but no gravitational waves.
Now, let's imagine Alice and Bob are so far apart that Bob is moving away from Alice faster than light (see 10 below). Alice starts out with very little mass, but decides to go off her diet. As a result, she gains an enormous amount of mass. So much so that she becomes a black hole (see equation 11). The distance between her and Bob is the same but it is now less than Alice's Scharzschild radius. So are Alice and Bob still diverging or are they now converging?
We know that no signal, limited to light speed, ever reached Bob. This includes gravitons, gravitational waves, light, etc. At 12, Alice's mass is converted to it's equivalent space. Finally, the inequality at 13 shows that Bob is still moving faster than light if Alice is at rest, but Alice is no longer at rest--she's moving faster than Bob towards Bob. Thus Alice and Bob are converging as if a "gravitational force" is present.
Take a proton and electron. Equation 14 below shows acceleration depends on both their respective charges, their masses and distance r. Clearly there is an information exchange between them. At equation 15 it's a different story: acceleration only depends on the mass of the proton and distance r. It is clear the two masses don't exchange information. It's as if the proton simply accelerates towards the electron.
Now, let's consider Alice, Bob and Carl below. How fast Alice accelerates depends on the distance of the targets, the targets being Bob and Carl. Surely Alice needs to know the distance of each target so she can adjust her rate of acceleration accordingly?
Consider the diagram below. The numbers in each section add up to the total volume of the 3X3X3 cube. At 17 the volume of each cube is divided by its square. If we multiply each term by the square of Hubble's parameter it becomes apparent that Alice is accelerating less than Bob and Bob is accelerating less than Carl. These three are diverging as if they are in an expanding universe.
Now, let's add mass to the red section. Let's convert it to its equivalent volume which is 100 units. That brings the total to 101 units. Notice that the state of Bob's section (blue) and the state of Carl's section (green) do not change. Each have their previous volume. Also notice that the square areas do not change. This implies no information exchange between Alice, Bob and Carl. But now Alice is accelerating more than Bob and Bob is accelerating more than Carl. The three are converging is if they are in a gravitational field.
The rate of acceleration depends on distance but, as demonstrated above, this does not imply an information exchange between the parties. To drive this point home, imagine we divide up our universe into volume cells, each cell expands at a given rate independently of every other cell. More cells cause a greater rate of expansion, but each cell has no clue how many cells an observer is looking at or what the other cells are doing. The cells exchange no information. Thus the rate of expansion is really up to the observer. Now, suppose you add more volume (cells) to the system without increasing the space. This was done when mass was added to Alice's section (red). Mass's equivalent volume doesn't change the distances between Alice, Bob, and Carl. The result is what we call gravitational acceleration (see equation 20 below). Again, each cell need not know the state of the others. The rate of acceleration depends on the distance the observer chooses to consider.
Now let's turn to quantizing gravity. The popular choice is, of course, the graviton, but the graviton causes a major setback. If we assume the graviton is a real thing, surely we can come up with a reasonable estimate of how many gravitons are in the universe. For example, we could figure the total gravitational energy of the universe and divide that by the average energy of the graviton. At 21 below we plug in the entire mass of the universe to get the gravitational energy, but we don't get units of energy. Instead, we get velocity squared. One could argue that there is no gravitational energy, so there are no gravitons. Equation 22 is an attempt to counter this argument. It uses two masses which give an energy term, but how much gravitational energy there is depends on how much of the universe's total mass we assign to m and m'. Also, whatever gravitational-energy total we arrive at will be exceeded by a single black hole singularity and a single particle that have virtually zero distance between them.
At equation 23 we switch to a smaller scale. We assume the gravitational energy produced by an electron and proton is nEg, where Eg is the energy of one graviton and n is the number of gravitons. Therefore two electron-proton pairs produce 2nEg or 2n gravitons? Not so fast. Since gravitons have energy, they also produce gravitons. At 24 they produce up to an infinite number of gravitons if the distance r limit is zero! So how many gravitons are in the universe? It depends on how you crunch the numbers.
By contrast, it is much easier to estimate how many photons are in the universe, since photons don't produce photons. Electromagnetic energy is a function of charge and not energy or mass. So as long as photons don't have charge, they don't infinitely reproduce themselves. We can take the total luminous energy of the universe and divide it by a photon's average energy to get a reasonable estimate of how many photons there are.
For the above reasons, the graviton is untenable. So then how should we quantize gravity? Gravity is a function of mass (defined as energy divided by light speed squared), space and time. Thus it would make sense to quantize these fundamental dimensions. We could ask, what is the shortest length or time, and, what is the smallest mass? The popular response is, the Planck length, the Planck time and the Planck mass, respectively. But are these really the smallest units? The Planck mass clearly is not the smallest mass. An electron mass is smaller. Also, the Scharzschild radius of an electron is much shorter than a Planck length. The time it takes for a photon to travel the shorter distance is less than the Planck time. So what are the smallest units? Here are the smallest units I have found so far. I call them the Hubble units:
We could take the Hubble length, for example, and quantize equation 13 as follows:
At equation 29 the first term has alpha Hubble lengths; the second term has beta Hubble lengths. The smallest rate of expansion is Hubble's parameter times one Hubble's length. Now, one probable objection to this scheme is space is chaotic and stochastic on the quantum scale, so how can we have such nice, neat units? The Hubble length, for example, could be an expectation value (average) of all the chaotic activity that may make up space. We can think of the Hubble units as perhaps the smallest average units that can be derived from fundamental constants and Hubble's parameter.
In conclusion, unlike the other fundamental interactions, gravity does not appear to have any means for bodies to communicate with each other, nor is communication necessary. Thus the graviton is unnecessary. Further, the graviton fails to conserve energy like its electromagnetic counterpart the photon. A better way to quantize gravity is to quantize space, time and/or mass.
Acknowledgments:
Amber Strunk. Education and Outreach Lead. LIGO Hanford Observatory.
Peter Laursen, Astrophysicist and science communicator at the Cosmic Dawn Center, University of Copenhagen.
References:
1. Parikh, Wilczek, Zahariade. 2020. The Noise of Gravitons. arxiv.org.
2. Feynman, R.P. 07/03/1963. Quantum Theory of Gravitation. Acta Physica Polonica. Vol. XXIV.
4. Carlip, S. 12/1999. Aberration and the Speed of Gravity. arxiv.org.
5. Van Raamsdonk, M. 05/17/2010. Building up spacetime with quantum entanglement. arxiv.org.
7. Gravitational Wave. Wikipedia.
8. de Rham, C., Tolley, A.J. 03/17/2020. Speed of Gravity. arxiv.org.
9. Carroll, S.M. 12/1997. Lecture Notes on General Relativity. Enrico Fermi Institute.
14. Nix, E. 08/22/2018. Who Determined the Speed of Light. History.com.
15. Speed of Gravity. Wikipedia.
16. Tests of General Relativity. Wikipedia.
17. Decross, M. et al. Gravitational Waves. Brilliant.com.
18. Lawden, D.F. 1982. Introduction to Tensor Calculus, Relativity and Cosmology. Dover Publications, Inc.
19. Stefanovich, E. V. 09/16/2018. A relativistic quantum theory of gravity. arxiv.org.
20. Light-time correction. Wikipedia.
21. Liénard–Wiechert potential Wikipedia.
23. Faber, J. A. 11/24/2018. The Speed of Gravity Has Not Been Measured From Time Delays. arxiv.org.
24. Yin Zhu. 08/18/2011. Measurement of the Speed of Gravity. arxiv.org.