According to all string theories, the Planck length and Planck time are the smallest units. In fact, the string is considered the smallest bit of matter. That brings us to the proverbial question: How long is a string? A string theorist will tell you it is the Planck length. The equations below show the components of the Planck units:
As you can see, the Planck units are made up of the following components: Newton's constant (G), Planck's constant (h-bar) and light speed (c). All these constants are believed to be constant. As long as they are, the Planck units are not just arbitrary units. On the other hand, if one or more of these constants are not always constant ... well that could be a problem.
If we begin with the Lorentz equation, we can derive equation 11 below:
If we assume nothing goes faster than light, then the change in time (delta-t) has an upper limit of t. Also, we know the following is true:
Thus, what follows is also true:
Now, let's assume black holes exist, look what happens when we let radius (r) fall to zero:
The light-speed barrier is broken and energy is no longer conserved. But, then again, if radius r is really small, Heisenberg's uncertainty principle might save the day.
Whoops! Nevermind. A particle, or black hole singularity in this case, can borrow energy from nothing but has to pay it back quickly, in time t. Since the black hole has to borrow more energy than is estimated for the entire universe, it's time is short indeed. The black hole, as we understand it, could not exist. Yet there is a black hole at the center of our galaxy--and it has been there much much longer than time t. How is that possible? Check this out:
If the constant G is allowed to shrink along with radius r, energy is conserved, so the black hole doesn't have to borrow--it can exist as long as it wants. Plus, the light-speed barrier is conserved as well. Unfortunately, the Planck units are arbitrary units. They are not constants of nature.
But does G really shrink? If so, when is it constant and when is it not? If we revisit equation 10, we can derive the following:
Equation 26 shows that delta-t has an upper limit of t but radius r has a lower limit of zero. Since these are components of G, G shrinks to zero as r shrinks to zero. By contrast, equation 27 shows that as r goes to infinity, delta-t goes to zero. As a result, G remains constant. So it appears G is constant at large distances. At small distances, G is no longer constant. For G to be constant at the quantum scale, delta-t needs to grow to infinity as r shrinks to zero. But if that were the case, energy would not be conserved:
Therefore, the gravitational constant G destroys much of modern physics if it remains constant at small scales, and, makes the Planck units arbitrary if it doesn't.
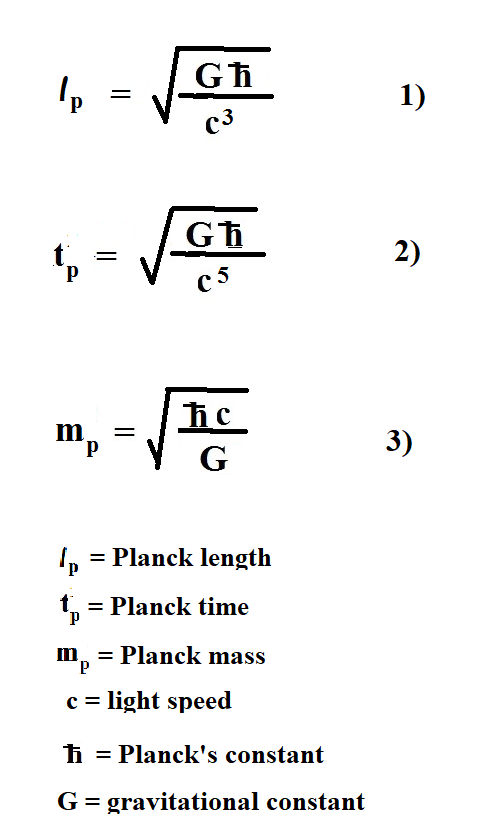









No comments:
Post a Comment